How To Add Or Subtract Scientific Notation
Abode / 8th Form / 6 Easy Steps for Adding and Subtracting in Scientific Notation
Hither's How to Add together and Subtract in Scientific Notation
When Adding and Subtracting in Scientific Note the exponents of the numbers you are adding or subtracting must be equal. If they are already equal, so you can but add the coefficients together. If the exponents are not equal, then you must make them equal by moving one of the decimals. The easiest way to make the decimals equal is to make the smaller exponent equal to the larger exponent by moving the decimal to the left. You add 1 to the exponent for each space that you move the decimal point to the left. Once the exponents are equal so yous tin can add or subtract the coefficients. The terminal stride for Adding and Subtracting in Scientific Notation is to ensure that the coefficient is between 1 and x. If it is not, you must move the decimal point so that it is.
Common Core Standard: 8.EE.A.3
Completing any Add and Subtract in Scientific Note Case Problem
When Adding and Subtracting in Scientific Notation the powers of the numbers you lot are adding or subtracting must be equivalent. In the issue that they are equivalent, yous tin just combine the coefficients together. If the powers are not equivalent, yous should brand them equivalent by moving one of the decimals so that the powers will get equivalent. The last step for Adding and Subtracting in Scientific Notation is to check that the coefficient is somewhere in the range of 1 and 10.
6 Quick Steps for Adding in Scientific Notation or Subtracting in Scientific Notation
- Check to run across if the exponents on the ability of tens are equal.
- If the exponents are equal, then you can add or decrease the coefficients.
- If the exponents are not equal, you must make them equal by moving the decimal bespeak.
- Moving a decimal point to the left will add to the exponent for each digit that it moves left.
- Once the exponents are equal, you can add together or decrease the coefficients.
- Make sure that your coefficient is in between i and x since it must be written in Scientific Notation.
Sentinel the Explanation of Adding and Subtracting in Scientific Notation Video
Sentry our free video on how to solve Calculation and Subtracting in Scientific Notation. This video shows how to solve problems that are on our free Adding and Subtracting in Scientific Notation worksheet that you tin become past submitting your email higher up.
Watch the free Adding and Subtracting in Scientific Notation video on YouTube here: Calculation and Subtracting in Scientific Notation
Video Transcript:
This video is well-nigh adding and subtracting in scientific notation. You can get the worksheet we apply in this video for costless by clicking on the link in the description below.
The beginning problem we're going to use to show y'all adding and subtracting scientific notation gives us 9 times 10 to the 9th minus 2 times ten to the 9th. When adding or subtracting in scientific annotation you are going to have the coefficients of each number that is being added or subtracted and you are going to either add or subtract those two together. In this case we have a 9 and a 2 these will be what we somewhen will add or decrease together. The second part of adding or subtracting a scientific notation that you need to be aware of is that our powers of 10 must exist equal. In other words the exponent on the power of ten must be the aforementioned. For example, if this was, let's say 10 to the seventh power, we could not decrease these considering this exponent is 9 and this exponent is 7. We would have to change the 7 into a 9 and once it was a 9 so we could add or subtract, merely in the case of our starting time example we have exponents that match.
So we're going to go ahead and solve this trouble. When solving this nosotros will take our coefficients which is nine and 2 and we're going to decrease them from each other, then we're going to rewrite our power of 10 right next to it. We will exercise times ten to the 9th power, when adding or subtracting the power of x has to be equal. One time the exponent is equal you lot will go on the exponent the same in your answer. The next step is to really go ahead and decrease the coefficient. 9 minus 2 is 7 and so we will rewrite times and then our power of 10 which is 10 to the 9th power. So our answer in scientific note is 7 times 10 to the 9th power considering nosotros practice non modify the power of 10 in our respond.
Number ii is very similar to number 1. We're given 3 six times ten to the seven plus two point 1 times ten to the 7th. We know we're adding at present we're going to add our coefficients together which is three point half dozen plus two indicate one. We're going to go ahead and write that 3 point half-dozen plus ii betoken one. Now if you lot wait at our power of ten our exponent is the same for each power of ten. 10 to the seventh here and x to the 7th here. We practice not accept to modify the exponent on the power of 10 because information technology is already equal, we will just rewrite 10 to the 7th power underneath of our problem. Then we're going to go alee and add our coefficients, iii betoken six plus two point i is 5 bespeak seven then we'll practice times 10 and and so nosotros'll bring down the seven. So our solution will be five point seven times 10 to the seventh ability.
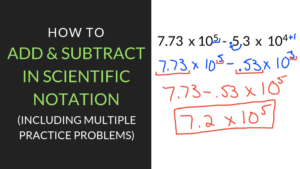
Number three is a little trickier considering it has i extra footstep we're given seven point seven three times ten to the fifth minus five indicate three times ten to the quaternary. Now in this example we cannot subtract these from each other because our exponents are not the same. You will notice this has a four and this has an exponent of five. They're non equal which ways we cannot subtract them we accept to alter one of them into the other and then once they are equal y'all can subtract them.
The easiest way to change the exponent on the ability of 10 is to ever make the smaller 1 into the larger one. What nosotros're going to do is we're going to change this iv into a 5. The way we do this is we motility the decimal to the left one and nosotros put it here and then we add one to our exponent. Every time you move the decimal to the left you will add one to the exponent. For example, let's say we movement the decimal instead of moving at one time we movement it i two times we would and so add two to this exponent. It would be 4 plus two but if we did iv plus ii that would be half dozen and we're non trying to make half-dozen we're trying to brand five so calculation ii doesn't brand sense. What nosotros're going to exercise is we're going to motility it left one time and and so we're going to add one because four plus one is 5 and we're trying to brand a five. Nosotros're trying to make the exponents equal then let's rewrite our problem.
Now we know that we accept seven signal seven iii on this side times x to the fifth power minus, and now on this side we have signal 5 3 considering we move the decimal left one time. 10 to the fifth ability because it's iv plus 1 which would be v. Now our exponents are equal, we accept a 5 here and nosotros have a v here then nosotros tin can go alee and decrease our coefficients. We'll exercise 7 bespeak seven 3 minus 0.53 and and then we'll rewrite times 10. And and then our power of ten which is ten to the fifth power because this is what we created to make them equal. And so we'll become ahead and subtract our coefficients, 7 signal seven 3 minus 0.53 will be 7 point two times 10 to the fifth power. And seven point ii times x to the fifth ability is going to exist our solution.
How To Add Or Subtract Scientific Notation,
Source: https://www.mathcation.com/adding-and-subtracting-in-scientific-notation/
Posted by: laughlininsing.blogspot.com

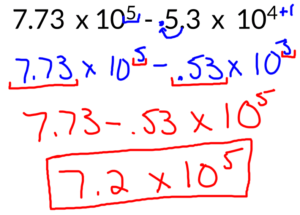

0 Response to "How To Add Or Subtract Scientific Notation"
Post a Comment